2000 AMC10 真题 答案 详解
| 序号 | 文件 | 说明 | ||
|---|---|---|---|---|
| 1 | 2000-amc10-paper-eng.pdf | 5 页 | 199.74KB | 真题-英文 |
| 2 | 2000-amc10-key.pdf | 1 页 | 9.93KB | 真题-答案 |
| 3 | 2000-amc10-solution-eng.pdf | 32 页 | 1.10MB | 真题-文字详解-英文 |
2000 AMC 10 Problems
Problem 1
In the year , the United States will host the International Math Olympiad. Let and be distinct positive integers such that the product . What is the largest possible sum ?
(A) 23
(B) 55
(C) 99
(E) 671
Problem 2
2000(20002000 ~ =
(A) 20002001
(B) 40002000
(C) 20004000
(D) 4,000, 0002000
(E) 20004,000,000
Problem 3
Each day, Jenny ate of the jellybeans that were in her jar at the beginning of that day. At the end of the second day, remained. How many jellybeans were in the jar originally?
(A) 40
(B) 50
(C) 55
(D) 60
(E) 75
Problem 4
Chandra pays an on-line service provider a fixed monthly fee plus an hourly charge for connect time. Her December bill was , but in January her bill was because she used twice as much connect time as in December. What is the fixed monthly fee?
(A) 82.53
(B) $5.06
(C) 86.24
(D) 87.42
(E) $8.77
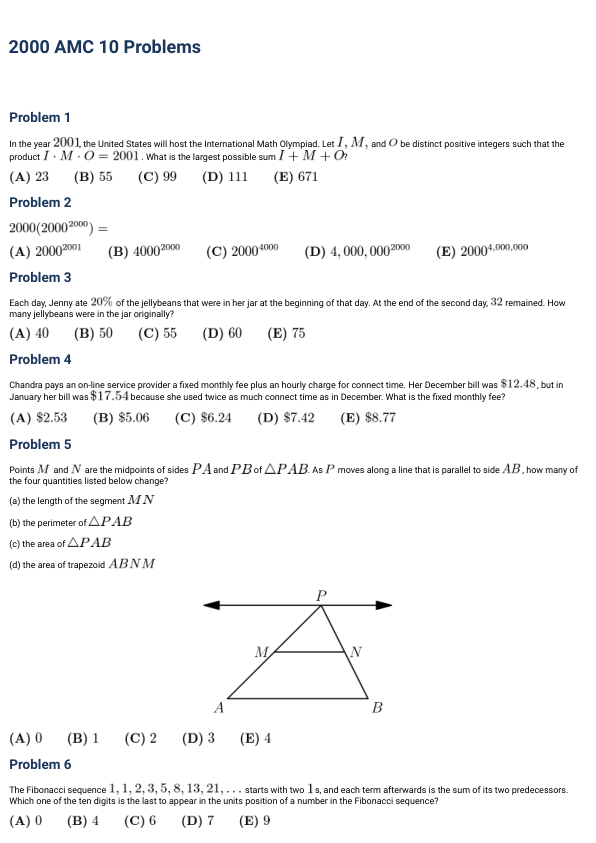
Problem 5
Points and are the midpoints of sides and of . As moves along a line that is parallel to side , how many of the four quantities listed below change?
- (a) the length of the segment
- (b) the perimeter of
- (c) the area of
- (d) the area of trapezoid
Problem 6
The Fibonacci sequence starts with two s, and each term afterwards is the sum of its two predecessors. Which one of the ten digits is the last to appear in the units position of a number in the Fibonacci sequence?
(B) 4
(C) 6
(D) 7
(E) 9
Problem1
In the year , the United States will host the International Mathematical Olympiad. Let and be distinct positive integers such that the product . What is the largest possible value of the sum ?
(A) 23
(B) 55
(C) 99
(D) 111
(E) 671
Solution 1 (Verifying the Statement)
667 + 3+1 = (E) 671_ First, we need to recognize that a number is going to be largest only if, of the factors, two of them are small. If we want to make sure that this is correct, we could test with a smaller number, like . It becomes much more clear that this is true, and in this situation, the value of would be . Now, we use this process on to get as our factors. Hence, we have
Solution 2
The sum is the highest if two factors are the lowest.
Solution 3 (Answer Choices)
(E) 671 We see since is divisible by , we can eliminate all of the first answer choices because they are way too small and get as our final answer.
Solution 4 (Faster Way)
= Notice , so we can just maximize this with , which has a sum of . Our answer is
(E) 671
.
