2001 AMC10 真题 答案 详解

---

---

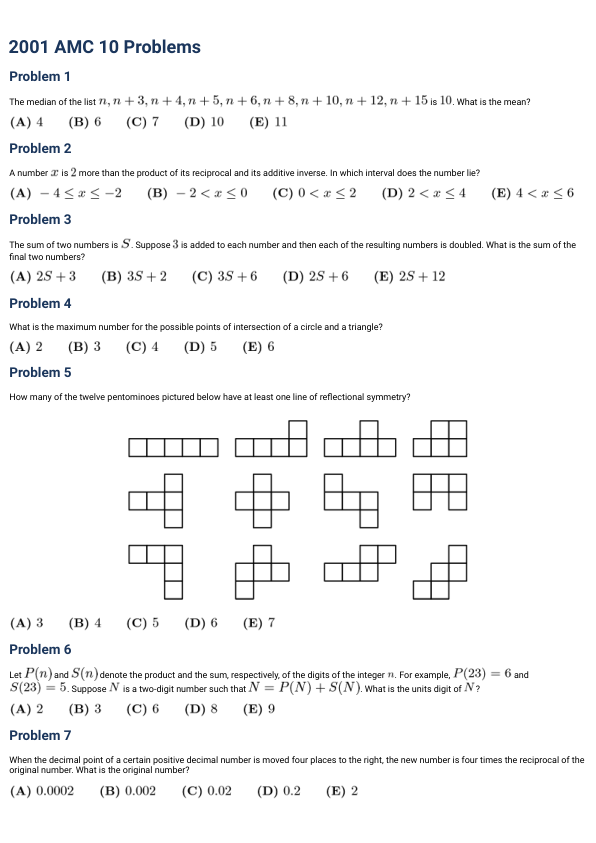
2001 AMC 10 Problems

Problem 1

The median of the list is . What is the mean?

(A) 2S + 3

(A) 2

(A) 3 (B) 4

(A) 0.0002

(A) 4

(A) = 4 < 2 < -2 (B) 2 < 2 < 0 (C) 0 < 2 < 2 (D) 2 < 2 < 4 (E) 4 < 2 < 6

(B) 3S + 2

(B) 3

(B) 0.002

(B) 6

(C) 3S + 6

(C) 4

(C) 5

(C) 0.02

(C) 7

A number is more than the product of its reciprocal and its additive inverse. In which interval does the number lie?

(D) 2S + 6

(D) 5

(D) 6

(D) 0.2

(D) 10

(E) 6

(E) 7

= Let and denote the product and the sum, respectively, of the digits of the integer . For example, and . Suppose is a two-digit number such that . What is the units digit of ?

(E) 2

(E) 11

The sum of two numbers is . Suppose is added to each number and then each of the resulting numbers is doubled. What is the sum of the final two numbers?

(A) 2

(C) 6

(D) 8

What is the maximum number for the possible points of intersection of a circle and a triangle?

(E) 9

How many of the twelve pentominoes pictured below have at least one line of reflectional symmetry?

When the decimal point of a certain positive decimal number is moved four places to the right, the new number is four times the reciprocal of the original number. What is the original number?

Problem 2

Problem 3

Problem 4

Problem 5

Problem 6

Problem 7

Problem1

The median of the list is . What is the mean?

(A) 4 (B) 6 (C) 7 (D) 10 (E) 11

Solution

The median of the list is , and there are numbers in the list, so the median must be the 5th number from the left, which is . We substitute the median for and the equation becomes .

Subtract both sides by 6 and we get .